The Triangle of Minimal Perimeter

By:

Alex Moore

Our goal in this exploration is to prove the following: Given an acute triangle ABC, the Orthic triangle is the inscribed triangle of minimal perimeter.

Proof: Let triangle ABC be any acute triangle, and let triangle DEF be any inscribed triangle in ABC. Let us first consider the placement of the point D on the segment AB. To help us “unfold” this problem, let us first reflect D across the line BC and across the line AC. Let D’ and D” denote the reflections of the point D.

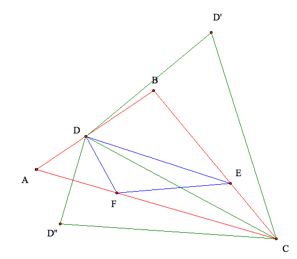
First notice that triangle D’ED is isosceles by definition of reflection and so the length of D’E is the same as the length of ED. For the same reason the length of DF is also equal to the length of D”F. Therefore, D’C = D”C. Since the perimeter of DEF is equal to DF+FE+ED, we now have the perimeter of DEF is equal to D”F+FE+ED’.

Using the old fact that the shortest distance between two points is a straight line, the perimeter of DEF will be minimize, for fixed position of D, when both E and F lie on D’D”, so we move E and F to lie on D’D”.

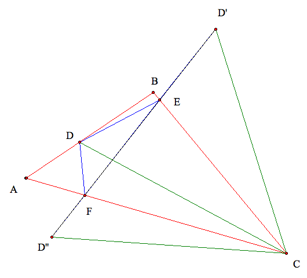
With E and F in place we now shift our attention back to D. Our next task is to position D such that DEF has minimal perimeter. Clearly moving D with alter the lengths of D’E and D”F, however, the angle D’CD” with remain at a constant value of twice the angle measure of angle BCA by properties of reflection. As D translates along AB the length of D’D” will change as well. Since the altitude from C to AB is the shortest distance from C to AB, moving D to this point yields the shortest length of CD, and therefore the shortest length of D’C and CD” as well due to reflection. Since triangle D’CD” is isosceles by reflection, this also gives the shortest length of D’D”. Therefore, Moving D to the intersection of AB with the altitude minimizes the perimeter of DEF since the perimeter has length D’D”. By the symmetry of the problem we can apply the same construction to E and F to see these points should also be at the foot of the altitudes.

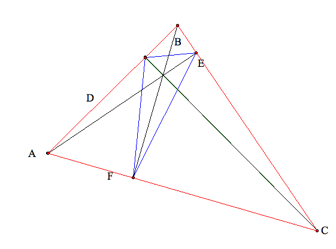
Therefore, the triangle inscribed in ABC of minimal perimeter is the Orthic triangle!

Now that we have proven the result for acute triangles, does this work for obtuse triangles? No! Why is that? If the triangle is obtuse then at least one of the altitudes is outside of the triangle and therefore at least one of the vertices is outside of the triangle. Hence the Othic triangle is not an inscribed triangle for obtuse triangles.

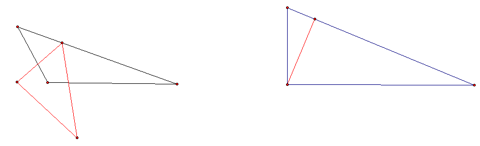
Does the result hold for right triangles? No! For right triangles the Orthic triangle is degenerate. Therefore, if triangle ABC is acute, then the Orthic triangle is the inscribed triangle of minimal perimeter.